The symmetrical universe

Warning: this post is devoid of contents.

During one of the very first classes of my Bachelor of Science in Physics, I got struck with a particular piece of information that sounded like a revelation to me:

If a problem exhibits a certain symmetry, the solutions to this problem do not necessarily exhibit that same symmetry, but the set of solutions always does.

The example my professor was using to illustrate this is the following. Imagine you have the four summits of a square and you want to find how to connect each point to all of the others using lines, but the total length of those lines must be as small as possible.

The problem itself has mirror symmetry, central symmetry and rotational symmetry with an angle of 90 degrees. In other words, the four summits are perfectly equivalent and can be exchanged without changing the problem in any way.

Now here’s the weird thing. There are two solutions, which are themselves less symmetrical than the problem itself and that look something like this: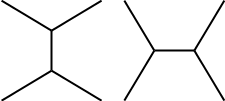

Notice how rotating any one of those by an angle of 90 degrees doesn’t keep it unchanged, but instead gives the other solution. In other words the set of solutions (those two solutions together) has exactly the same symmetries as the problem itself although each one of them separately doesn’t.

This is more far reaching than it seems and is nothing else than the phenomenon called spontaneous symmetry breaking that has been keeping a good number of physicists busy during the 20th century and the beginning of the 21st. Because there is only one universe that we can observe, whenever a physical process has the same characteristics as the above problem, the physical world has to choose one of the solutions and “break” the symmetry of the problem.

Now in any physical process, the experimental conditions are never perfect and small perturbations are likely to push the system to this or that particular solution so there is nothing super-weird about what’s happening here.

But there are phenomena where an external nudge to the system can’t explain the symmetry breaking: the ones that are at the origin of the universe. Cosmologists are actually still debating explanations to why the universe is so dissymmetrical (the arrow of time, the four fundamental forces, the inhomogeneity of matter, etc.) whereas the equations that seem to describe it are so symmetrical.

Many plausible explanations do exist, such as that our observable universe is part of a bigger “multiverse”. The weaker versions of this idea still consider a connected symmetrical universe that is one big multidimensional space-time continuum with different local regions where symmetry is broken in all possible ways. But why do we need the connectedness at all? Is it even an option? If you look at the square problem above, those two solutions are entirely disconnected and you couldn’t find a connected symmetrical solution if you tried. Why would the multiverse then need to be connected?

This leads us to the strongest version of the multiverse concept, the Mathematical Universe Hypothesis. The idea behind this is to attribute reality to all mathematical structures and to postulate that our observable universe is just one of this infinite number of structures (in an interesting case of taking the map for the territory).

Mathematical structures have this interesting property that they exist independently of culture, the human mind and even physics. Group theory for example could be discovered in any universe and would yield the exact same list of finite groups. In other words, they have lots of the qualities we associate with the reality of our own physical universe. Going from that to the idea that our physical world is just emerging from that primordial mathematical soup is quite tempting.

Now of course, it has been objected that such an idea is not testable or falsifiable and thus cannot be called scientific. That is absolutely true. But it does have that Occam’s razor quality of simplifying some of the apparent complexity of our universe. It also has the advantage of being an entirely naturalistic hypothesis to the origin of the universe if you’re into that sort of thing.

All this to give you an idea of the rush of ideas that went through my 20 year old brain at the precise moment when that professor showed us those two simple diagrams that you see above, oblivious at the time that those ideas that seemed so new and original to me had actually already been invented and debated a couple of years earlier. I felt at this instant the raw explanatory power of science and also its ability to extend its influence way beyond its self-imposed limits of testability. It is without a doubt the most powerful instrument of thinking and the best catalyst of ideas that the human mind has invented.